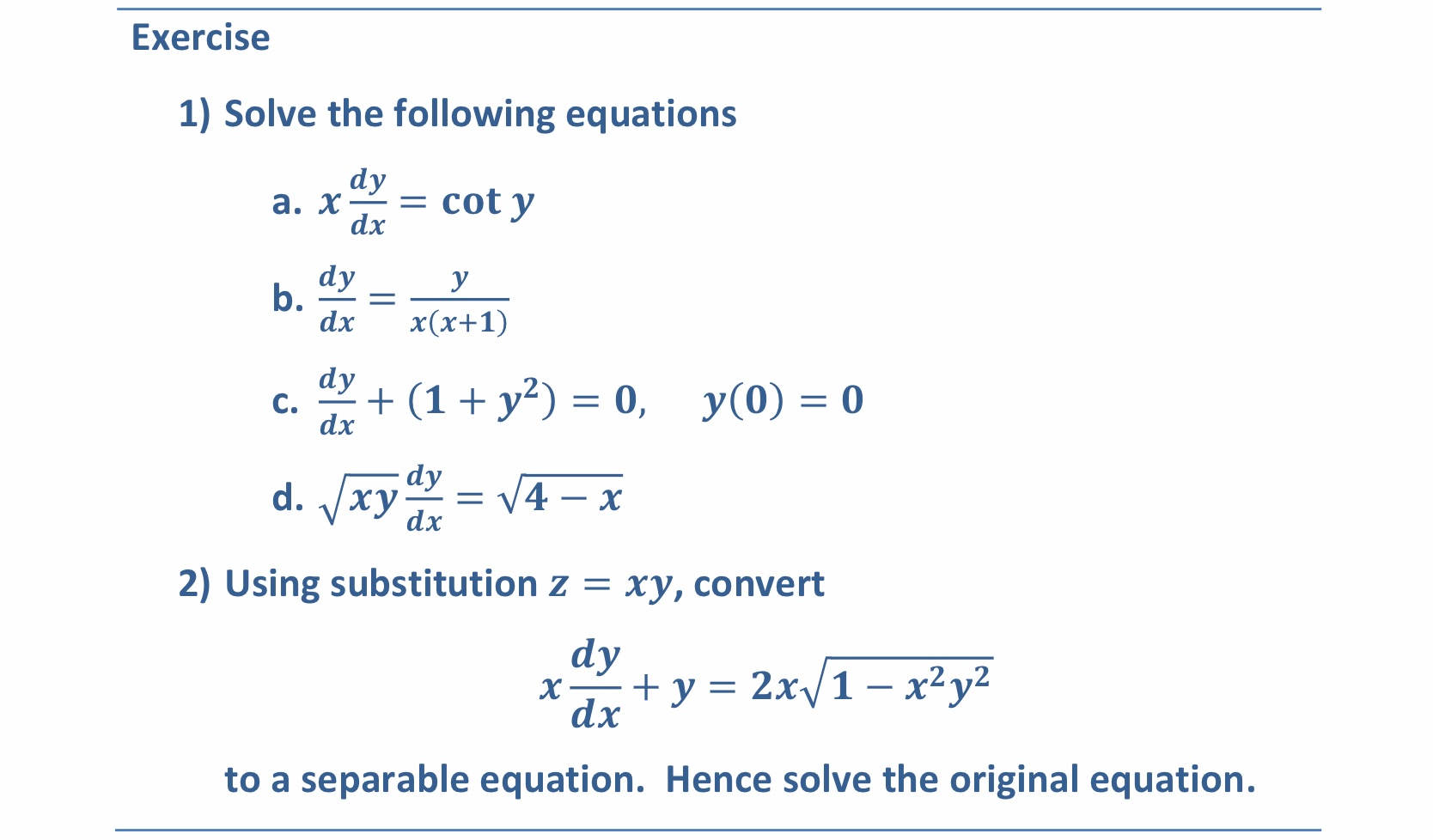
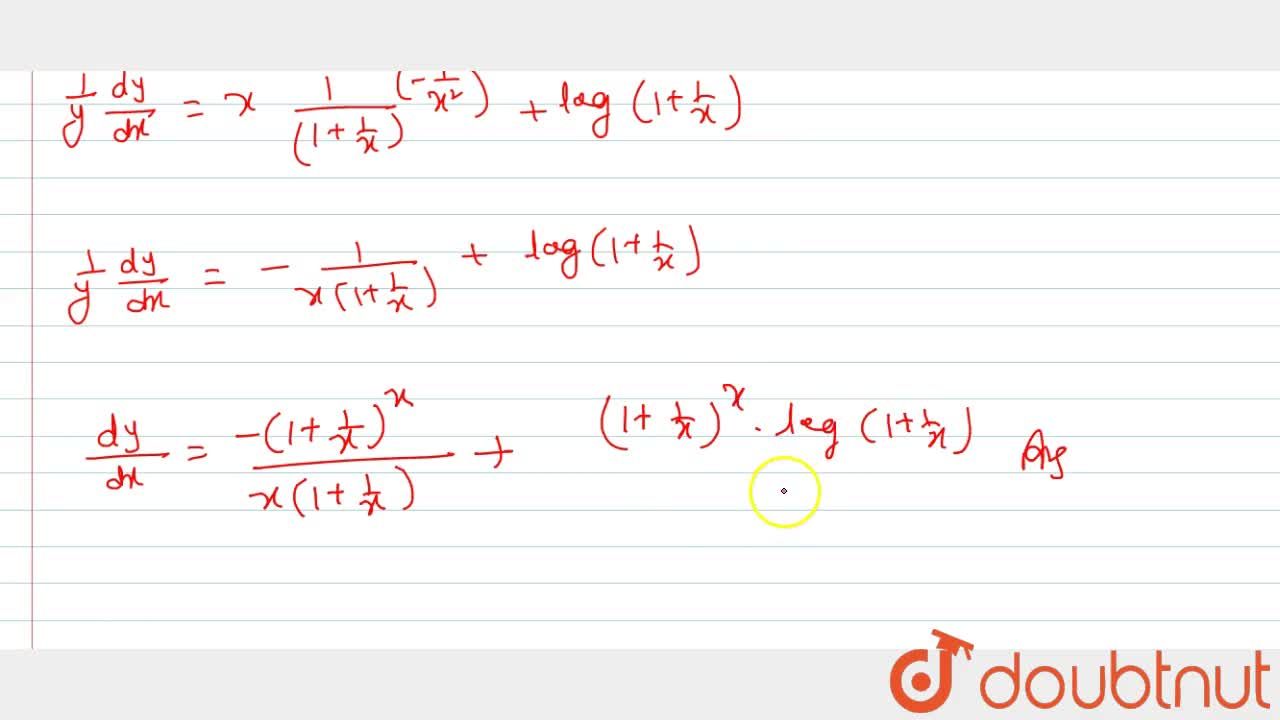
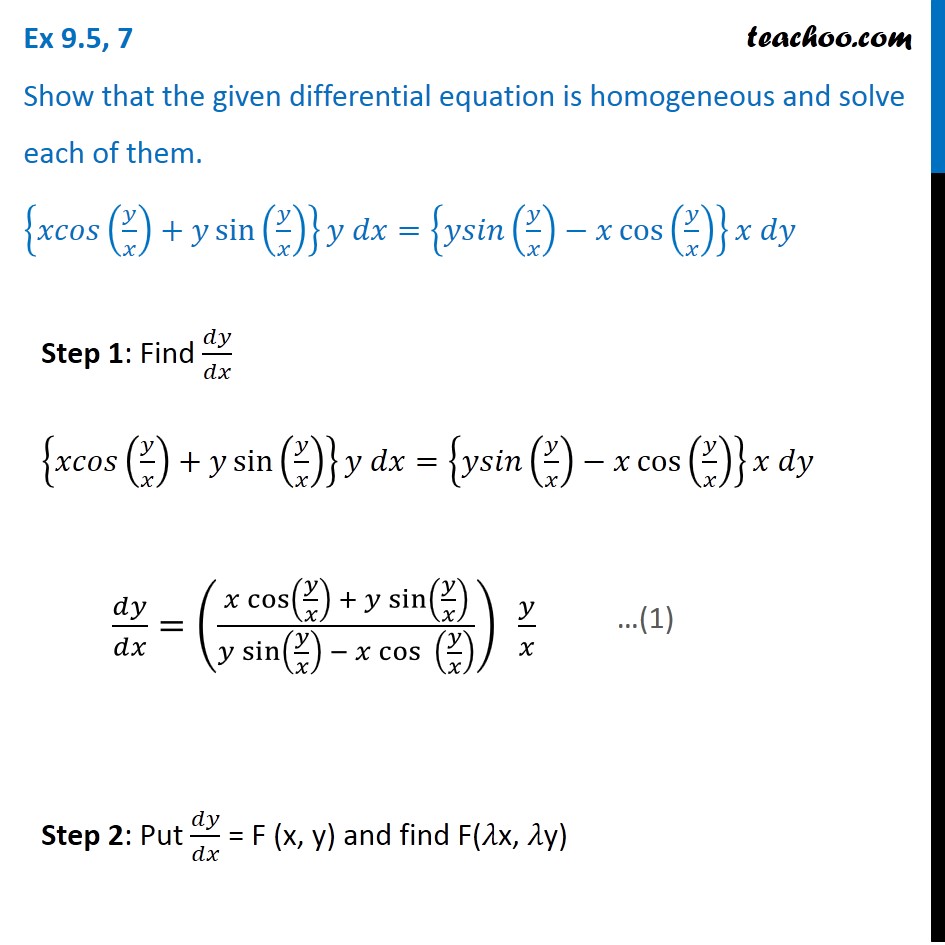
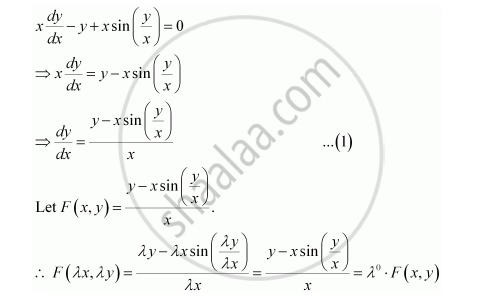
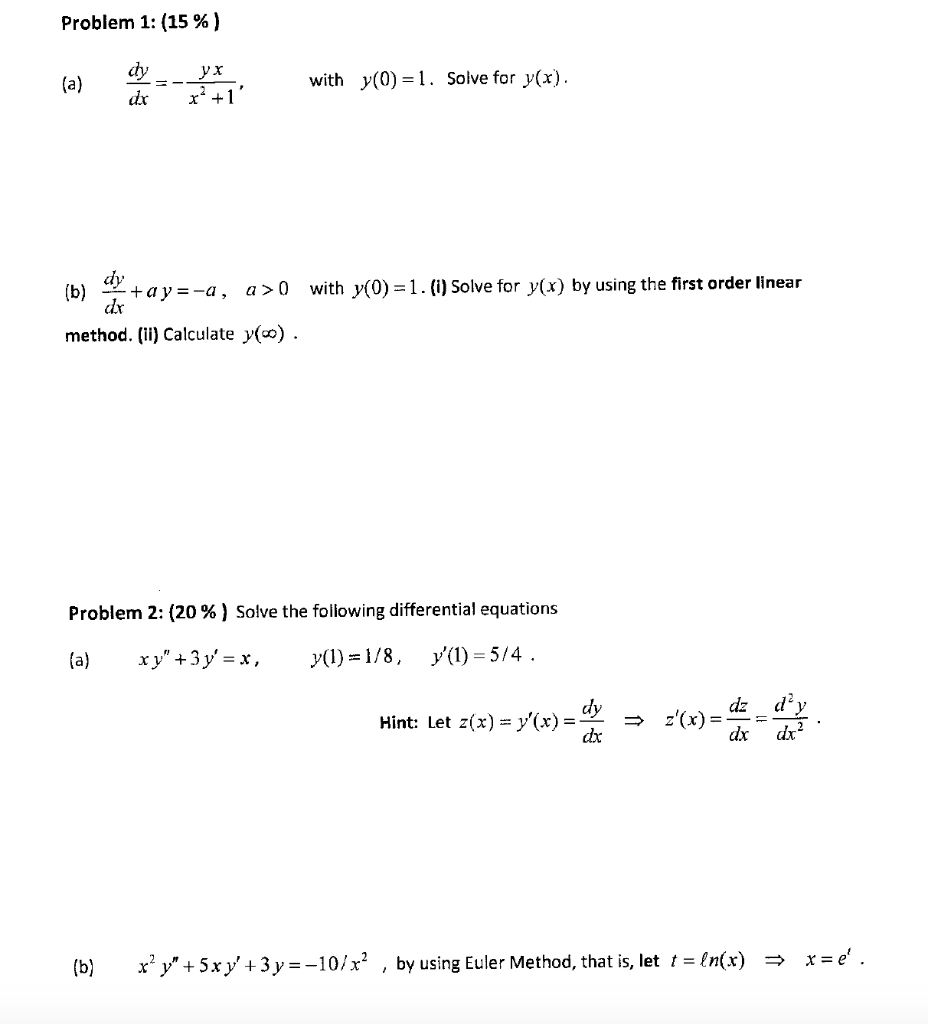
Find dy/dx of the functions
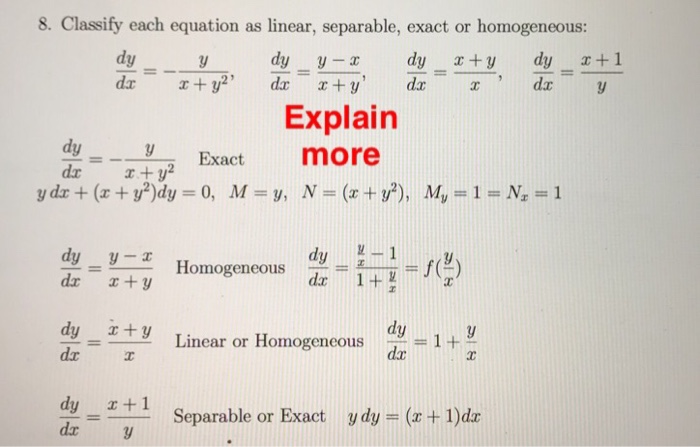
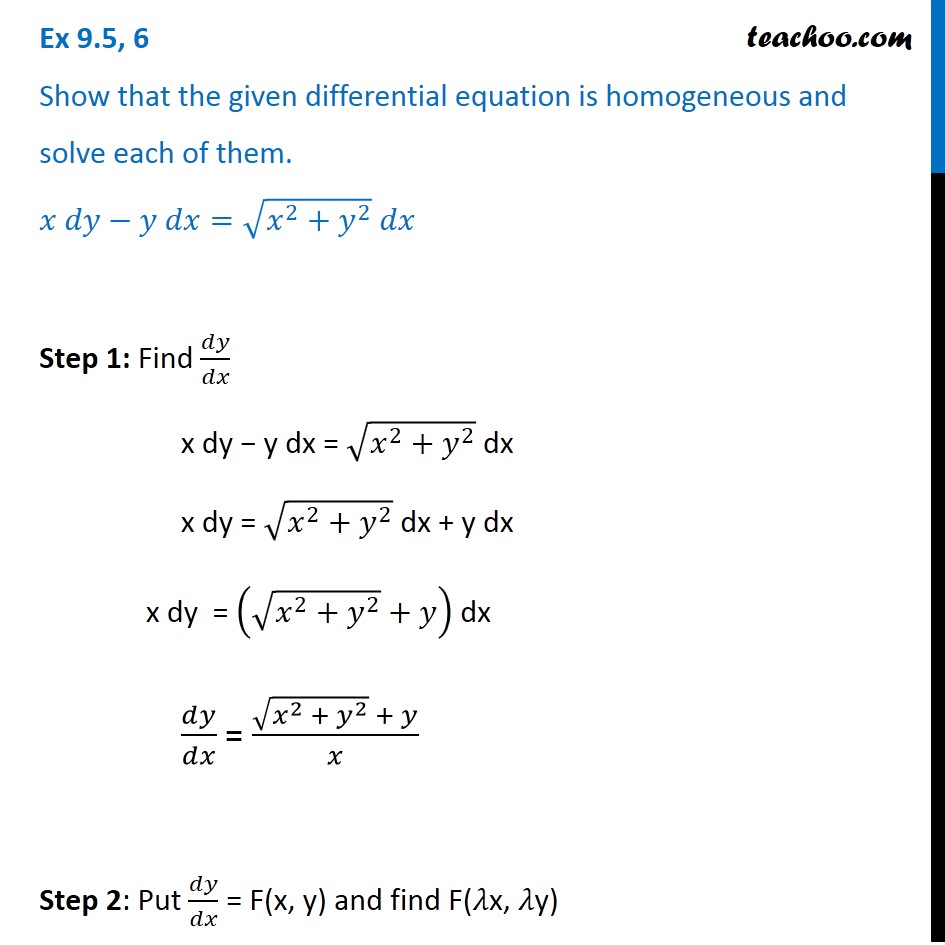
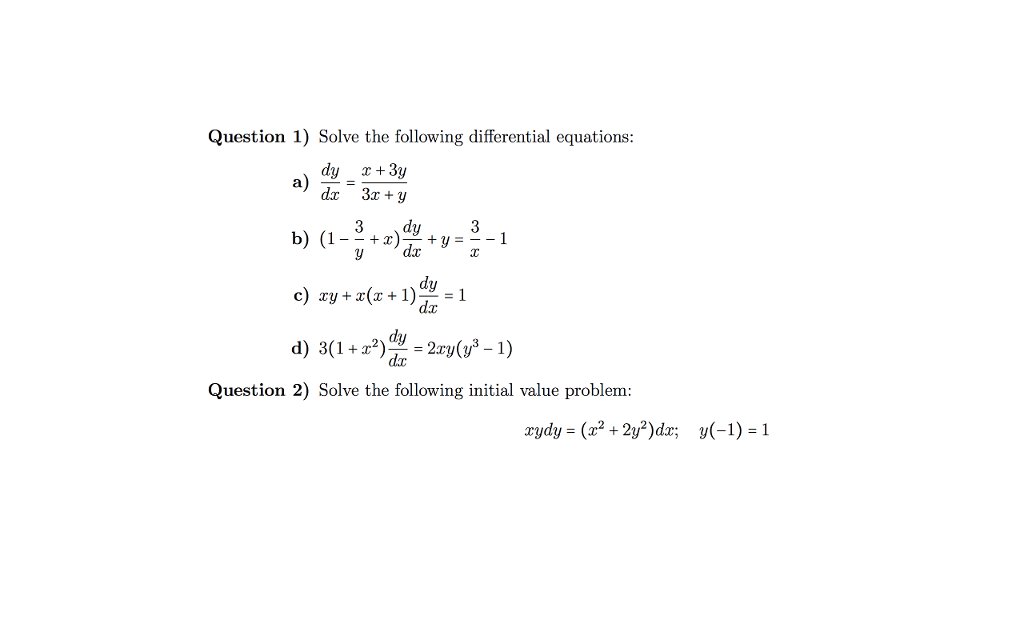
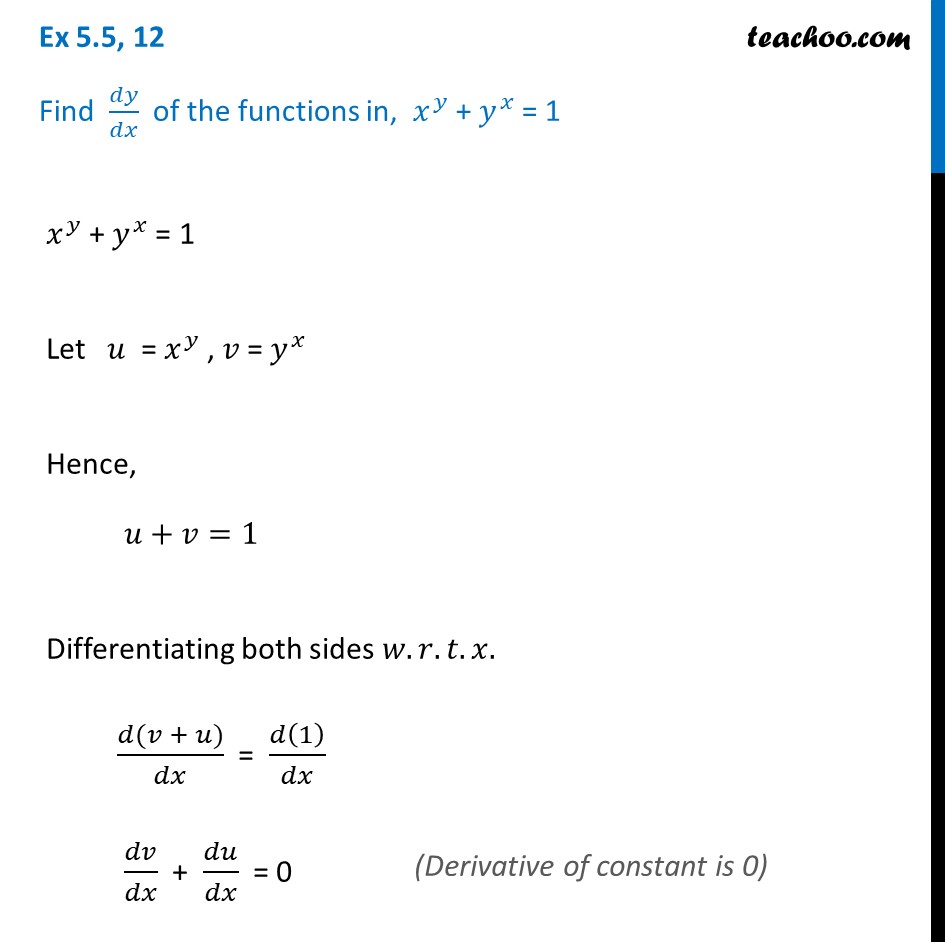
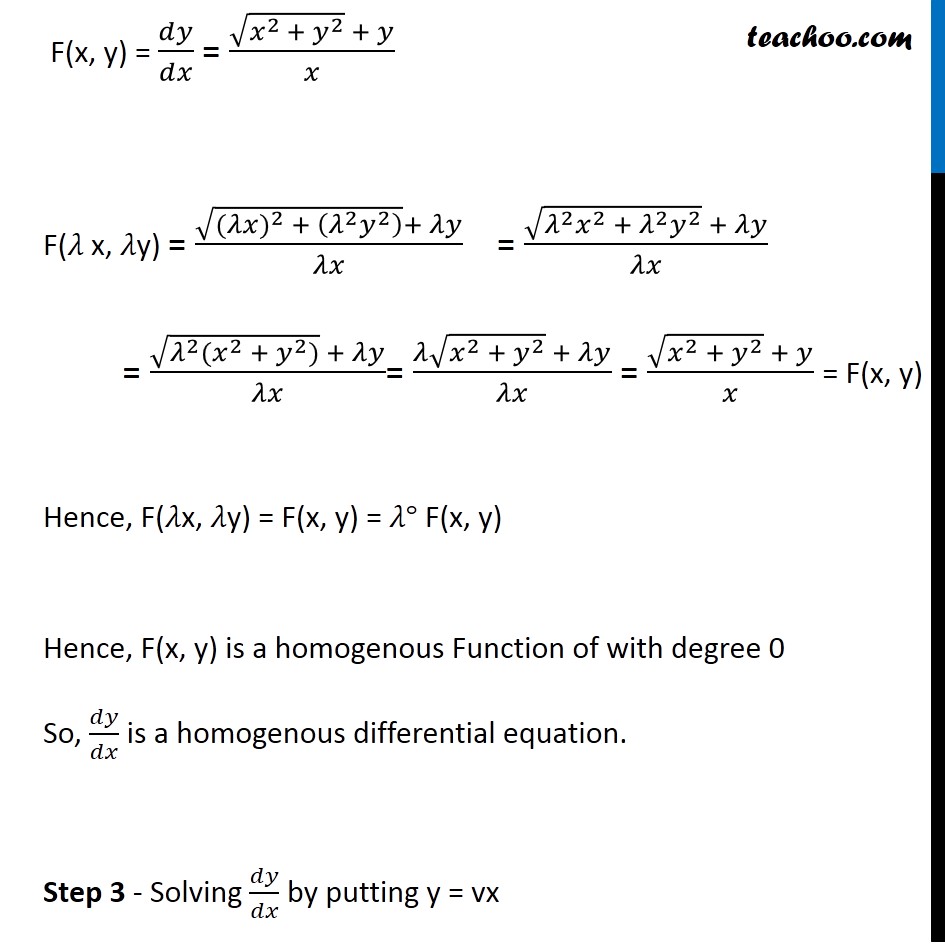
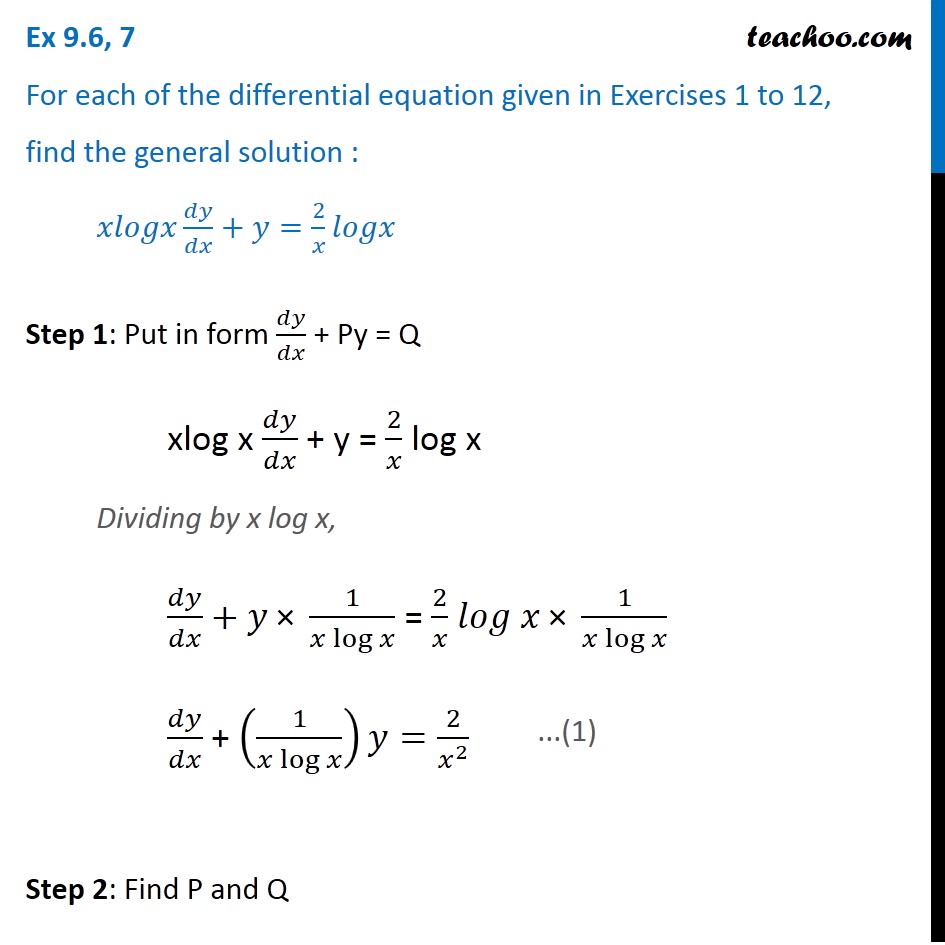
Y=x/x+1 find dy/dx- Ex 96, 3 For each of the differential equation given in Exercises 1 to 12, find the general solution = 2 = 2 Differential equation is of the form = where P = 1 and Q = x2 Finding integrating factor, IF = e IF = e Suppose that, y=x^(x^x) lny=(x^x)lnx ln(lny)=ln{(x^x)lnx}=ln(x^x)ln(lnx), ie, ln(lny)=xlnxln(lnx) Diffing both sides wrt x, d/dx{ln(lny)}=d/dx{xlnxln(lnx)}(star) Here, by the Chain Rule, d/dx{ln(lny)}=1/lny*d/dx{lny}=1/lny*d/dy{lny}*dy/dx ;
Y=x/x+1 find dy/dxのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 | ||
 |  |  |
 |  | |
 |  |  |
「Y=x/x+1 find dy/dx」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 | ||
 |  |  |
「Y=x/x+1 find dy/dx」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | 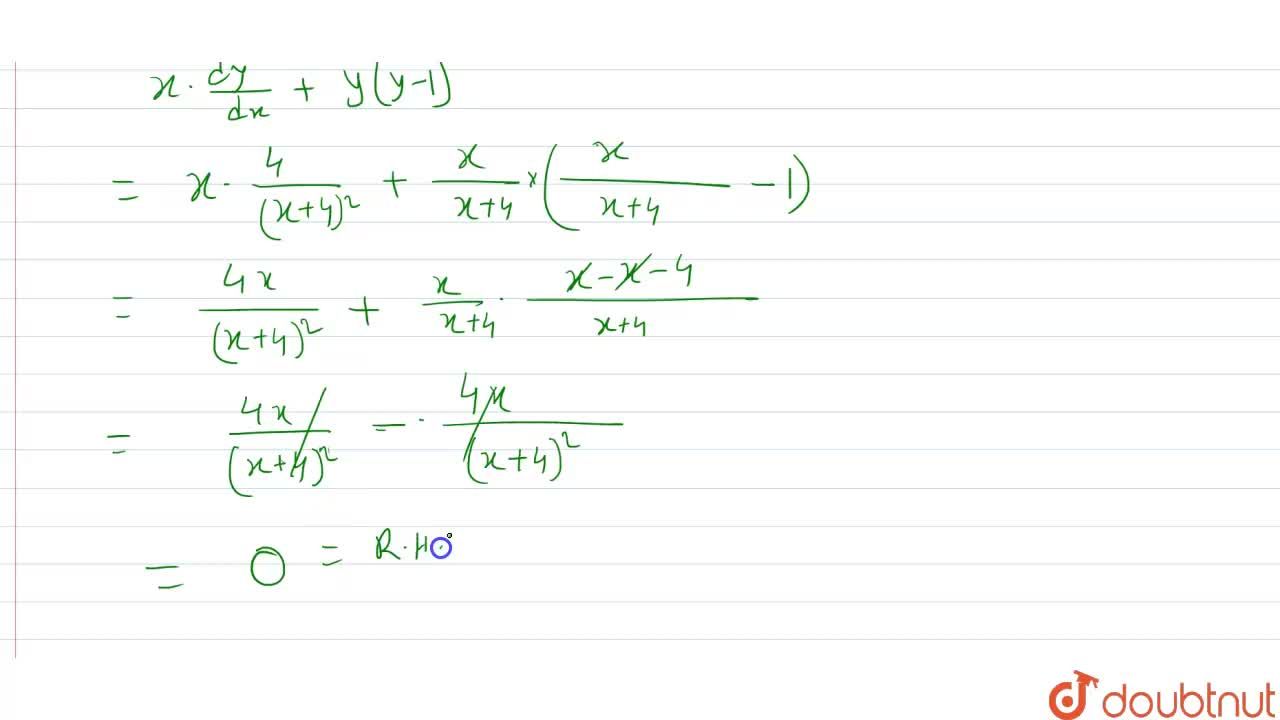 |
 |  |  |
 |  | |
「Y=x/x+1 find dy/dx」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  | |
 |  |  |
「Y=x/x+1 find dy/dx」の画像ギャラリー、詳細は各画像をクリックしてください。
 | 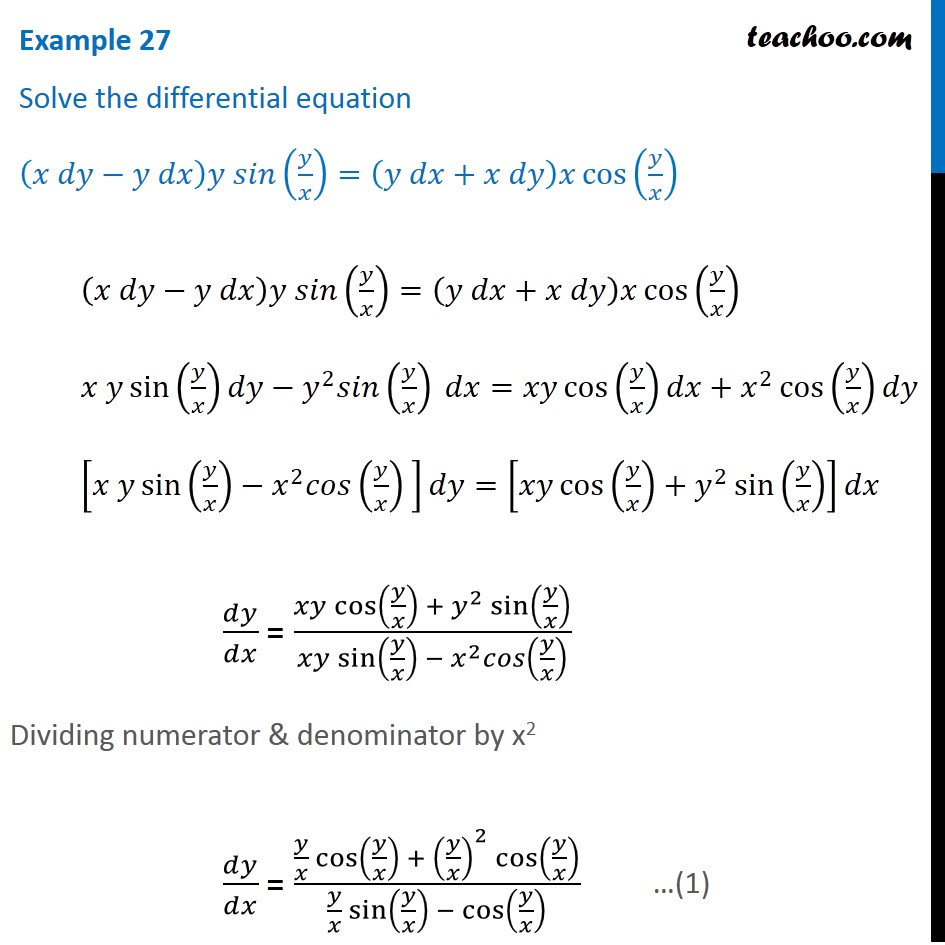 |  |
 | ||
 | 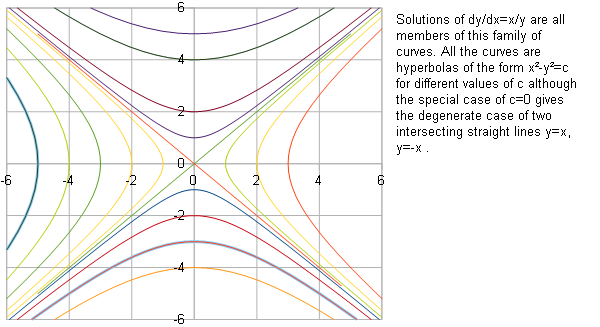 |  |
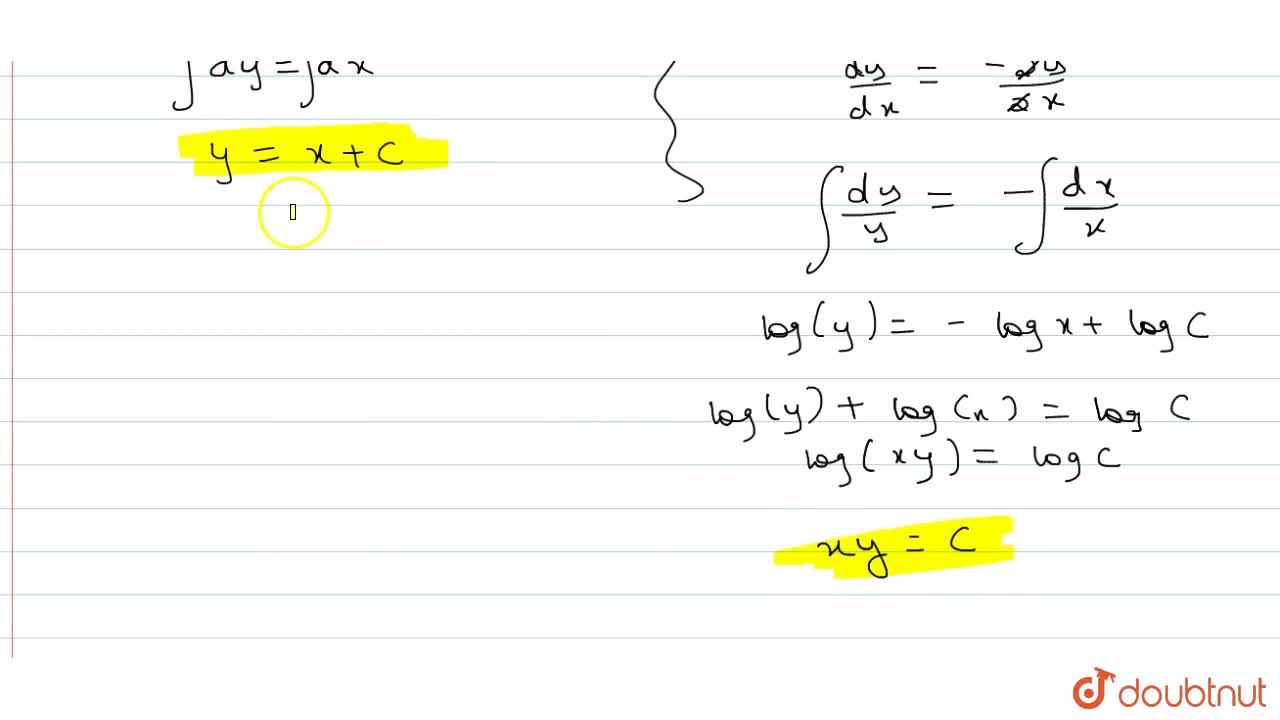 |  |  |
「Y=x/x+1 find dy/dx」の画像ギャラリー、詳細は各画像をクリックしてください。
 | 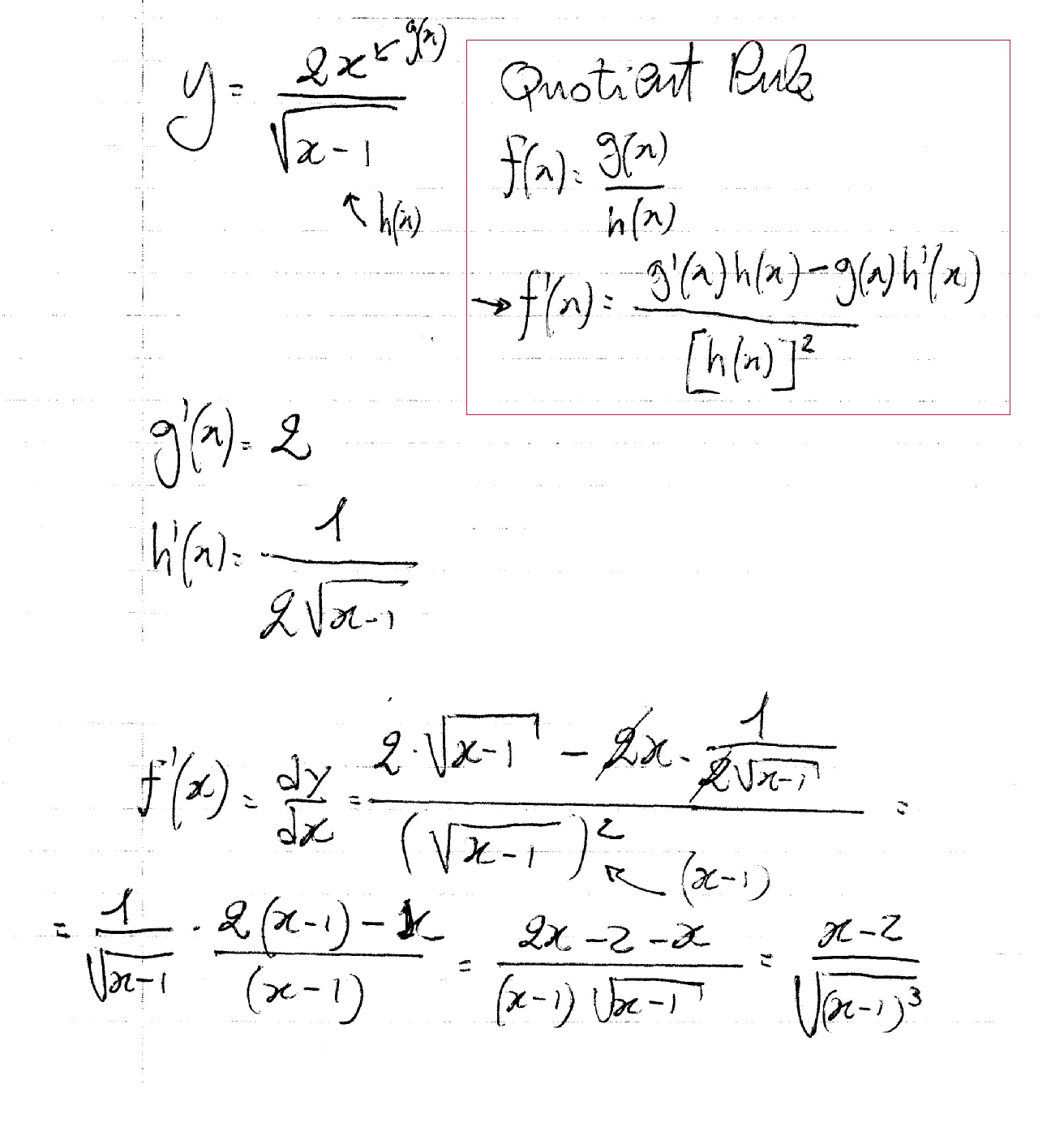 | |
 |  | |
 |  |  |
 |  | 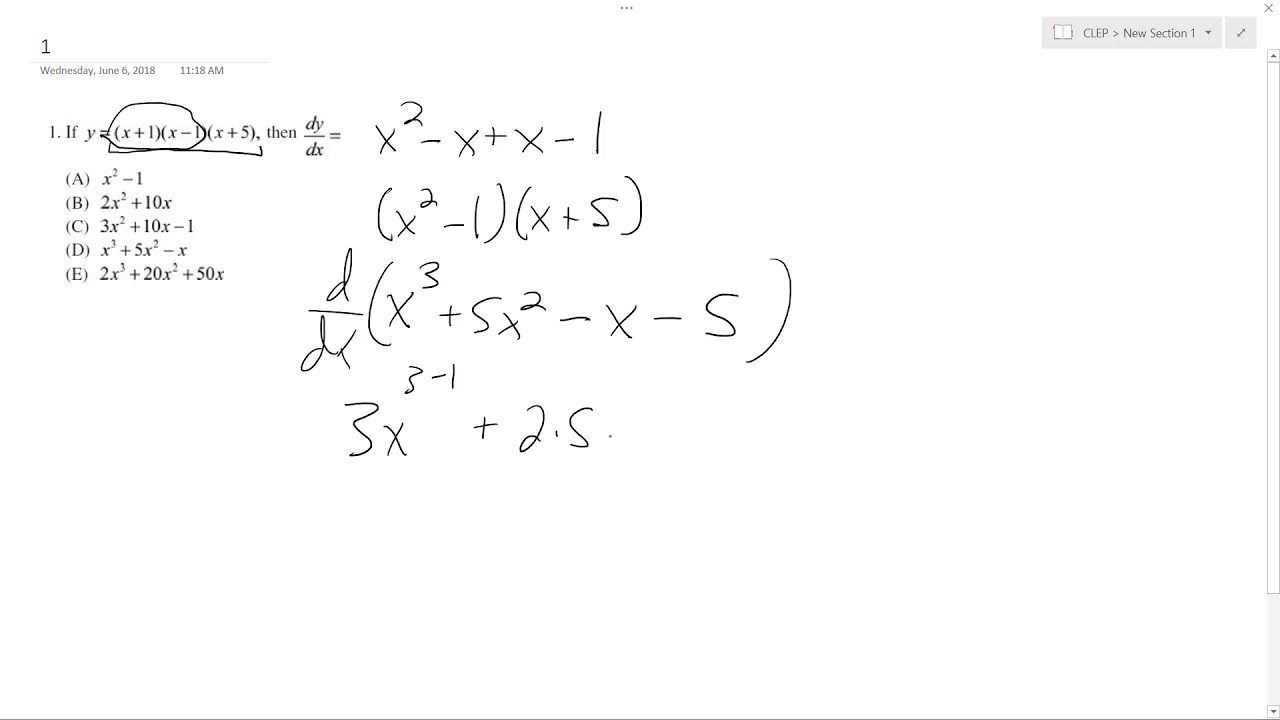 |
「Y=x/x+1 find dy/dx」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
 | 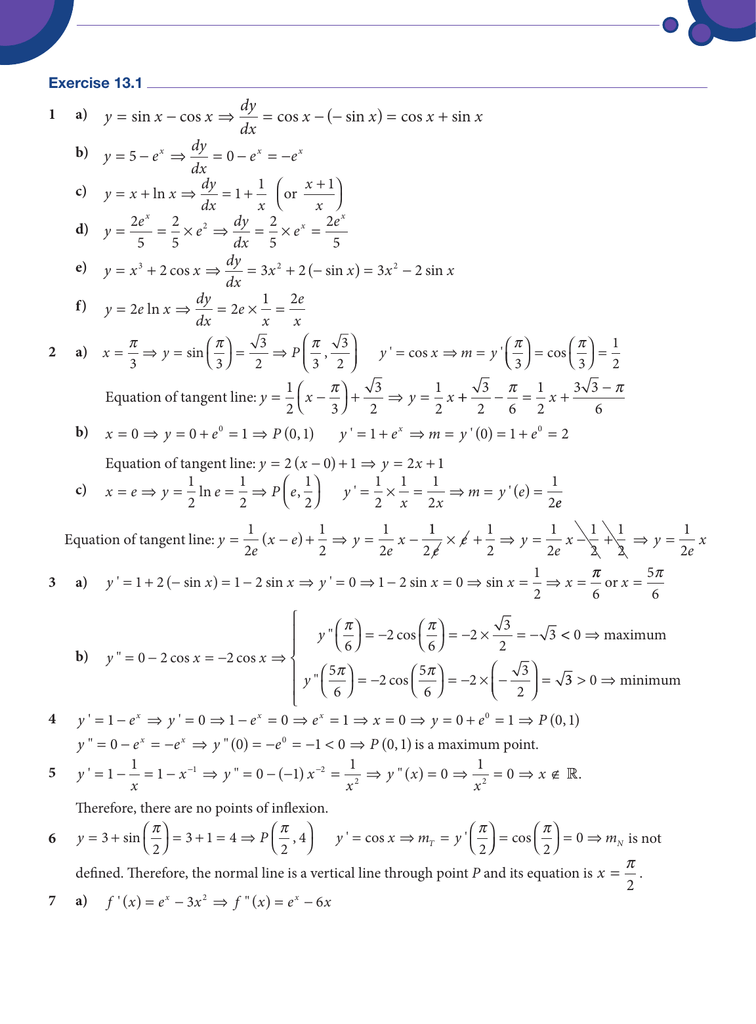 | |
「Y=x/x+1 find dy/dx」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
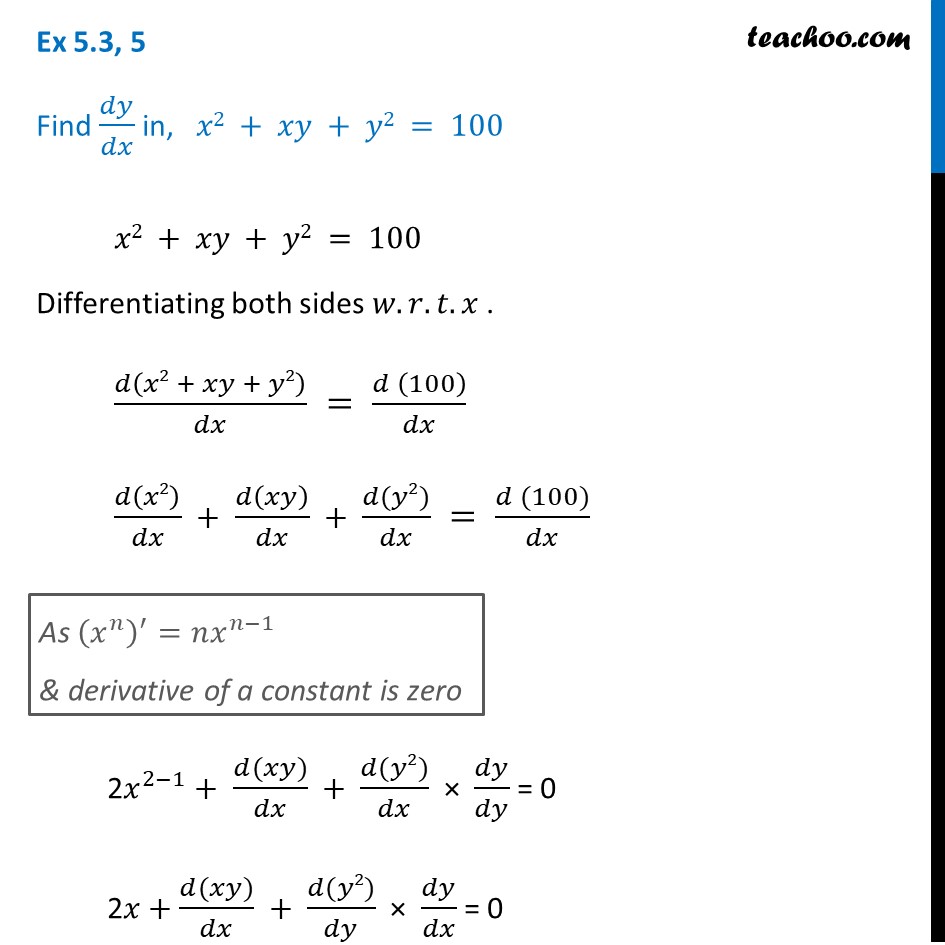
 | 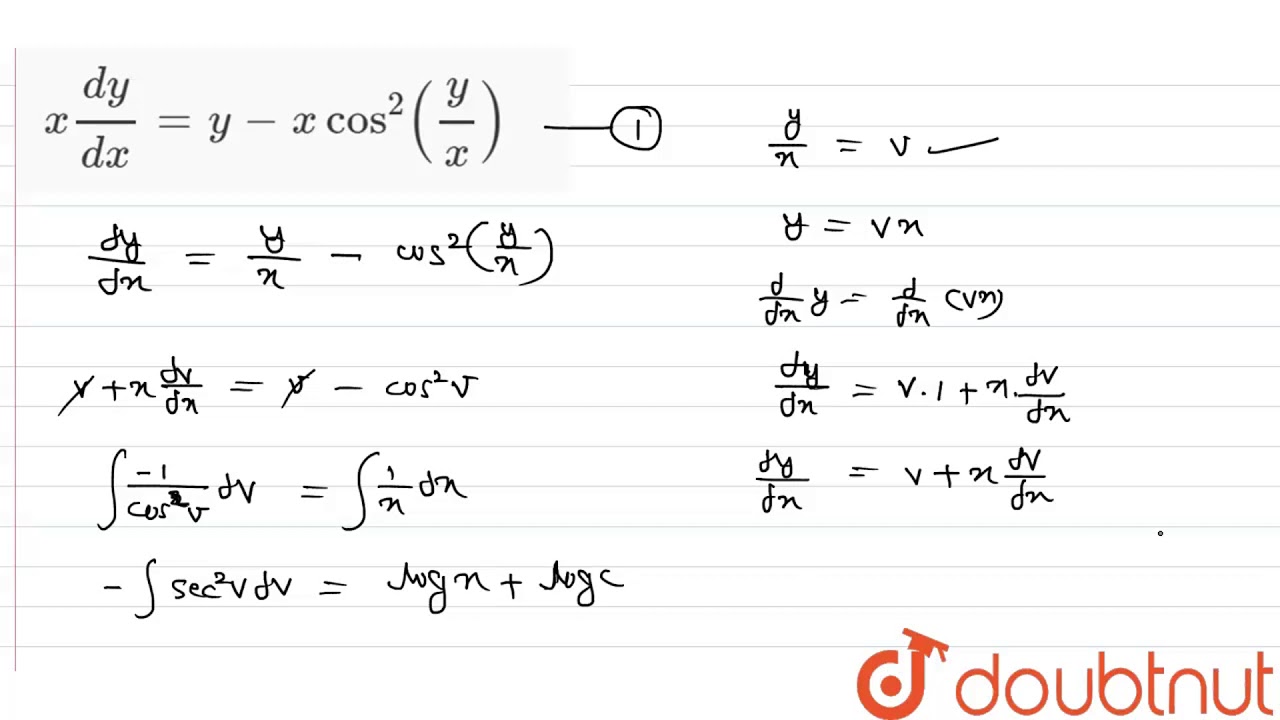 |  |
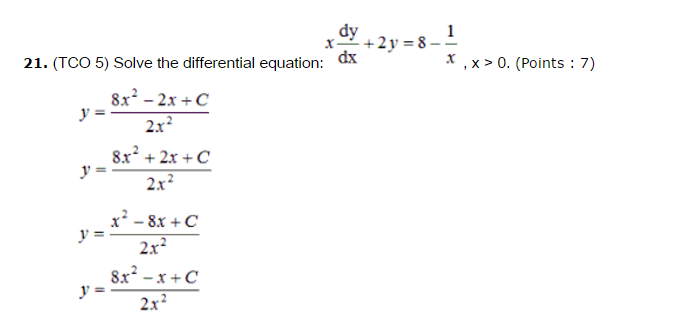 | ||
「Y=x/x+1 find dy/dx」の画像ギャラリー、詳細は各画像をクリックしてください。
 | 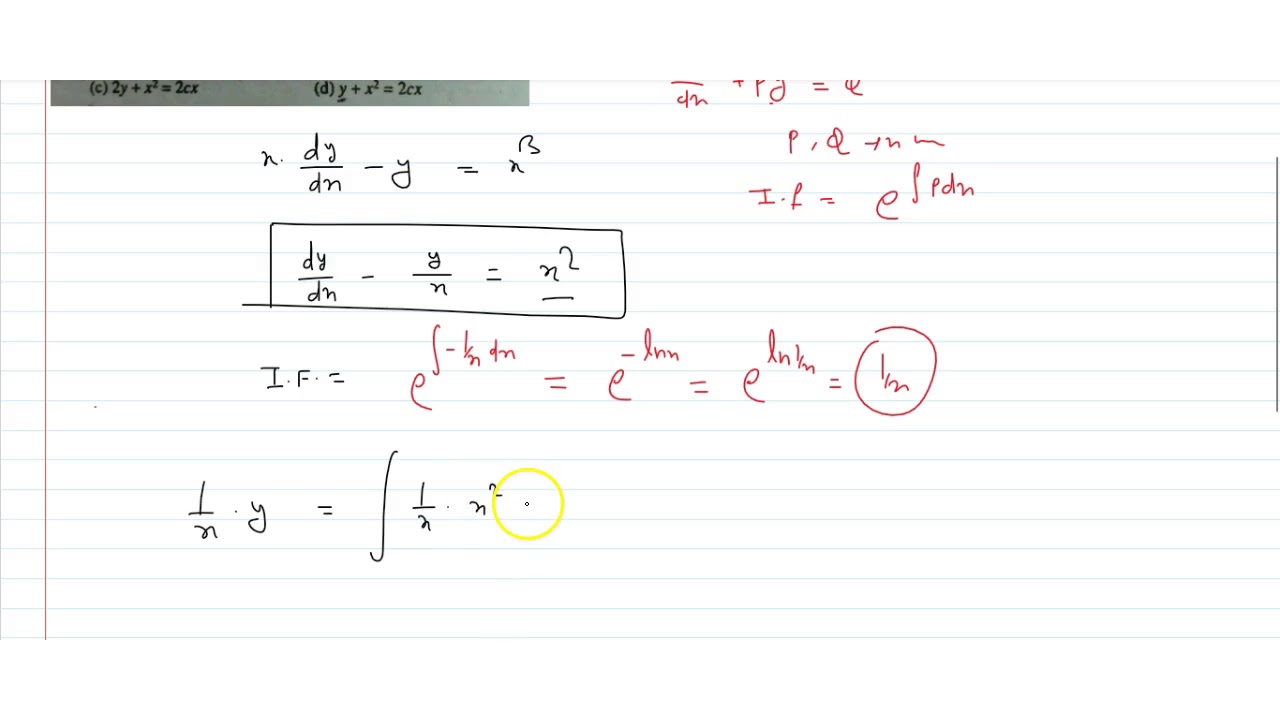 | 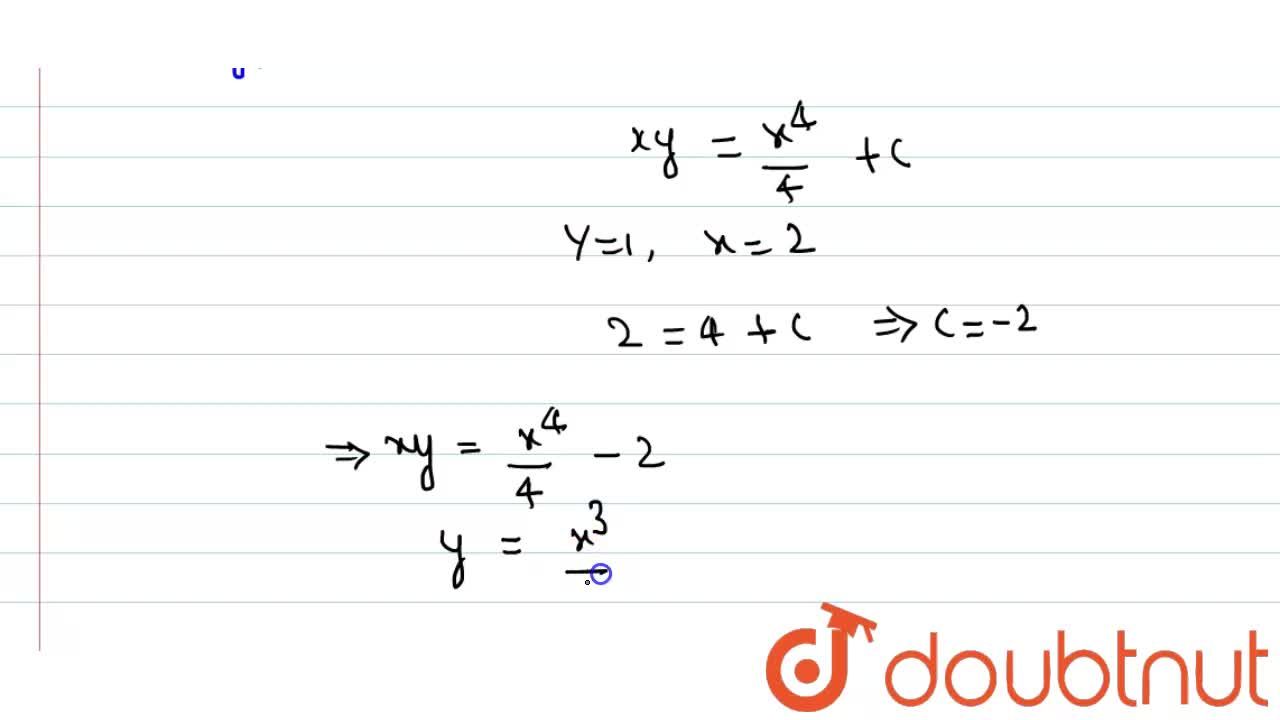 |
 | ||
 |
(dy)/(dx)=x^x(1lnx) we can use logarithmic differentiation d/(dx)(x^x) let y=x^x take natural logs of both sides lny=xlnx we now differentiate wrt" "x the 'LHS will be need the chain rule, the RHS the product rule d/(dx)(lny)=d/(dx)(xlnx) 1/y(dy)/(dx)=lnxd/(dx)(x)xd/(dx)(lnx) 1/y(dy)/(dx)=lnxxxx1/x (dy)/(dx)=y(1lnx) (dy)/(dx)=x^x(1lnx)D x d y = x ( y − x) d y Multiply both sides of the equation by x\left (xy\right) Multiply both sides of the equation by x ( − x y) x\left (xy\right)\frac {\mathrm {d} (y)} {\mathrm {d}x}=dy x ( − x y) d x d ( y) = d y Use the distributive property to multiply x by xy
Incoming Term: y=x^x dy/dx=, x^y=e^x-y dy/dx, y=x^x find dy/dx, y=sin(x^x) dy/dx, y=x^x then dy/dx, (x+y/x)dx-dy=0, y=x^x^2 then dy/dx, y=x/x+1 find dy/dx, tan(x+y)=x dy/dx, if y=x^x then dy/dx is,




0 件のコメント:
コメントを投稿